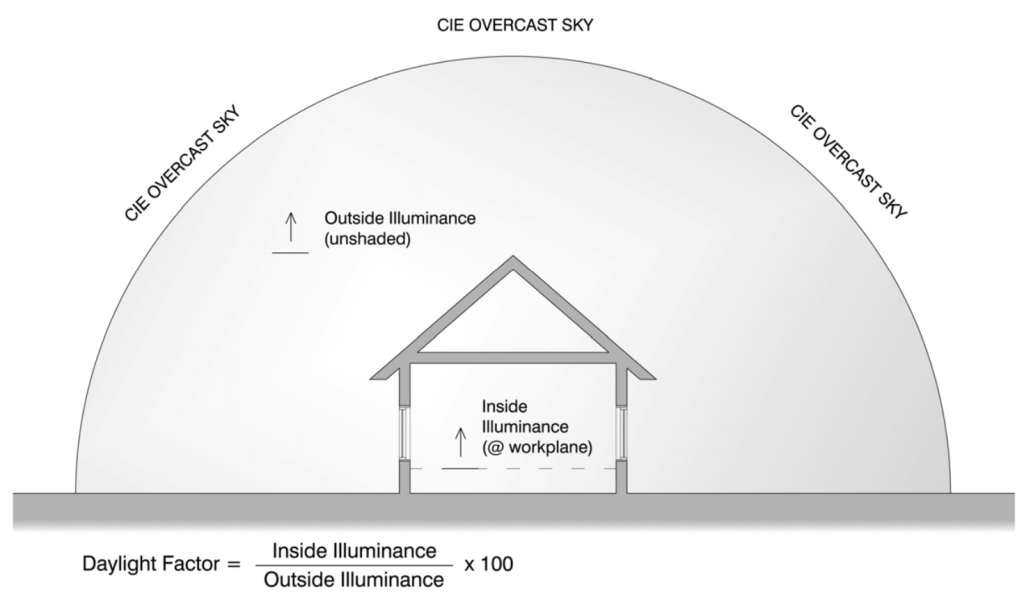
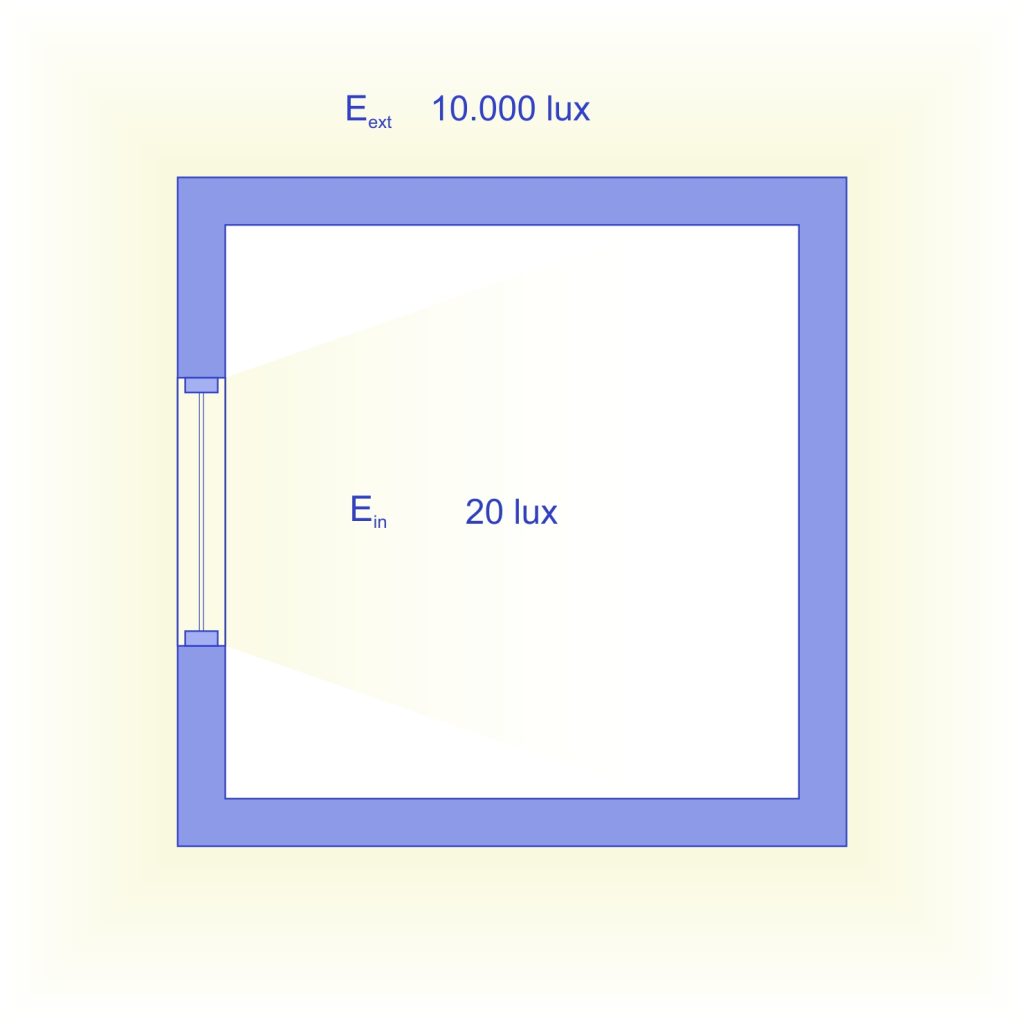
1. Daylight Factor
Daylight factor (DF) is defined as the ratio of interior illuminance to outdoor illuminance at the same time under an overcast sky condition. The DF is a commonly used measure for calculating the subjective daylight quality and availability in a room. The higher the DF, the more natural light is available in the room. The DF is depended upon the latitude; hence, DF is different for a same type of building constructed at different locations due to a change in outdoor illuminance.
It is expressed as,
DF = (Ein/Eext ) × 100
Ein : Interior illuminance at a fixed point on the workplane.
Eext : Exterior illuminance under an overcast sky.
The ‘mean daylight factor’ of a room is the average daylight factor value of a grid of sensors at work plane height that extends across the room.
According to the British Standards Institution, BS 8206 part 2 CIBSE a space with an mean daylight factor between 2% and 5% is considered well lit and requires little or no additional lighting during daytime. A space with a daylight factor of less than 2% appears dimly lit. Some examples of daylight factor requirements in different space types are listed below:
Office/Retail 2%
Classroom/Conference Room 3%
Circulation Area 1%
2. Window to Floor area Ratio (WFR)
The window area in daylighting refers to the proportion of window space relative to the floor area of a room, which significantly impacts both natural light availability and energy efficiency. This ratio, often termed the Window to Floor Area Ratio (WFR), is crucial for optimizing daylighting in architectural design. The formula for WFR is:
WFR = Window Area (WA) / Floor Area (FA)
A well-calibrated WFR can lead to significant energy savings, as larger window areas can decrease electricity consumption when integrated with artificial lighting and hybrid ventilation systems.
A common rule of thumb suggests a WFR of 20% to 25% for effective daylighting (Hayman et al., 2009).
Studies indicate that the ideal window area varies based on room geometry and orientation, with simulations showing that optimal sizes can differ significantly across climates. The effectiveness of the WFR can vary with latitude, necessitating adjustments in different regions to achieve optimal daylighting (Hayman et al., 2009).

Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
3. Window to Wall area Ratio (WWR)
The amount of light entering into a building is dependent on the ‘window-to-wall area ratio’. It is the ratio of the area of non-opaque building envelope components of dwelling units to the envelope area (excluding roof) of dwelling units. The greater the window-to-wall ratio (WWR %) more the light penetrates through the openings.
WWR = Area of Opening/Window / Total Wall Area
(excluding mullions and window frames) (width × floor to ceiling height)
A common rule of thumb suggests a maximum WWR of 40%, as outlined by ASHRAE Standard 90.1, which serves as a guideline for vertical fenestration area relative to wall area (Kahsay et al., 2020). However, optimal WWR can vary based on specific building characteristics and environmental conditions. A range of 20% to 25% is often cited for effective daylighting, though its applicability varies by latitude (Hayman et al., 2009).
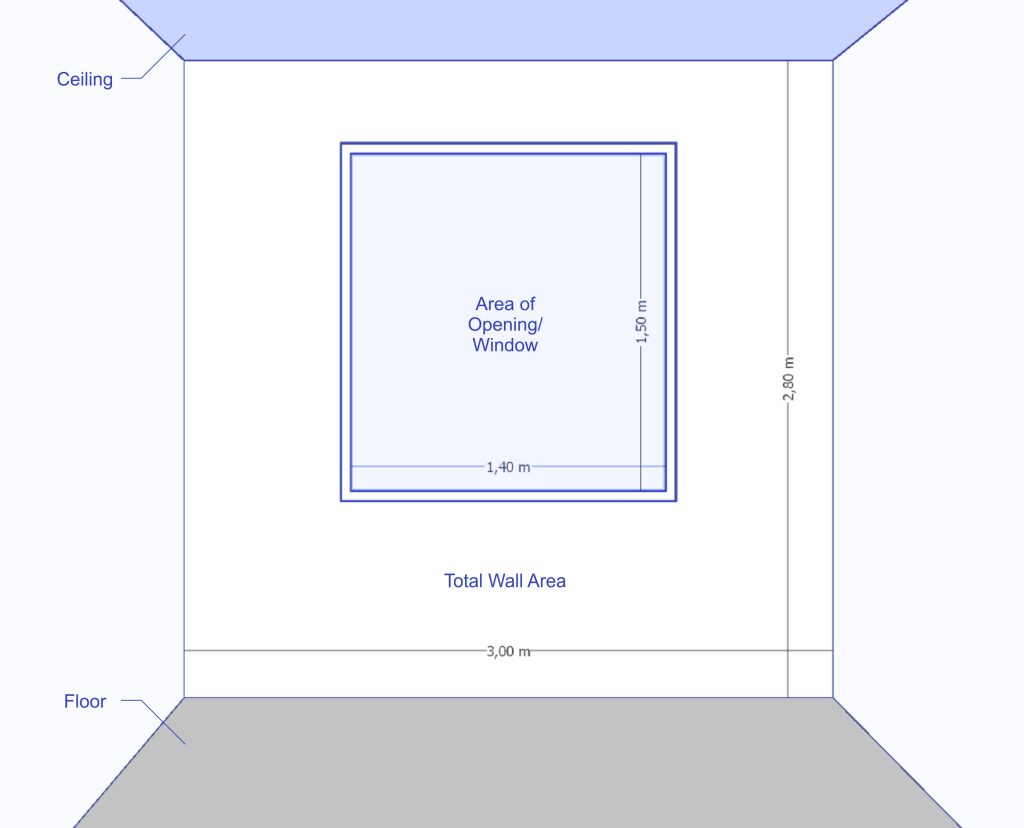
Example of WWR 25%
4. Limiting Depth
These are popular rules of thumb appearing in a wide range of sources including textbooks with some restrictions. Three factors that limit room depth should be considered in daylight design:
a) Daylight Uniformity
Distance at which the uniformity of daylighting levels throughout the space drops. The mean daylight factor becomes a poor representation of the daylighting levels in spaces under overcast sky conditions in the case of deep rooms, since they have very high daylight levels near the windows and very low values at the rear.
The maximum acceptable depth of a space as far as daylight uniformity is concerned can be approximated by:
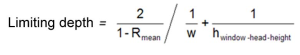
Rmean : Mean surface reflectance W : Room width in meters
Spaces with depths lower than this with depths lower than this limiting depth limiting depth usually exhibit relatively usually exhibit relatively uniform levels of daylighting throughout.
b) No Sky Line Depth
Distance away from the windows at which the sky is no longer visible. The room depth (at the height of the working plane) past which there is no direct view of the sky. It is defined as:
![]()
Where, α (No sky line angle) ~ θ (Sky angle)
Staying within this limiting depth allows for a space whose work surfaces all ‘see’ asas least some part of the sky least some part of the sky.
Note: α = θ is an approximation and is only valid if Δheight (top of obstructing building – centre of window) is substantially larger than Δheight (window head – centre of window).
c) Depth Of Daylight Area
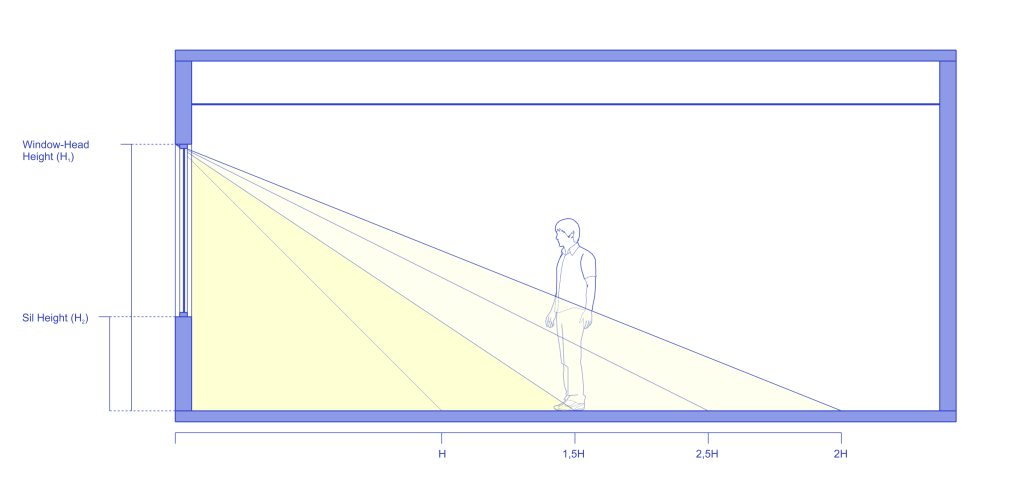
Distance to which ‘meaningful’ levels of daylight extend throughout the space. Daylight penetration in a space varies linearly with window head height. The relationship factor varies depending on whether or not a shading device is used.
Graduate School of Design, Harvard University suggested the following formulas (Otis, T & Reinhart, C., 2009):
With no shading device:
Limiting depth (H) = 2.5 . Hwindow-head height
With a shading device:
Limiting depth (H) = 2.0 . Hwindow-head height

Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.

Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
Other source (Malhotra, R., 2021) said, the depth of daylight zone is typically 1.5–2 times the window head height. If a space does not require the use of a shading device, the ratio range can increase up to 2.5-times.
Meanwhile online source states that the maximum depth of daylight entering a space is 2.5 times the difference between lintel height (H1) and task or sill height (H2), i.e.,
Depth of Daylight (H) = 2.5 . H1 (Lintel Height) – H2 (Task or Sill Height)
Source: Link
Depth of Daylight Rule of Thumb